Attempts to debunk my "Moderna and Pfizer are kill shots" post fail badly

I'll summarize the arguments and show you the data so you can decide for yourself

Executive summary

Several attacks have surfaced attempting to find a flaw in my Moderna/Pfizer analysis that I published yesterday.

I’ll show you in this article why all fall flat.

Also, the attackers are not calling for data transparency. They also attempt to change the topic when confronted with questions they find uncomfortable.

Generally, the approach is create fear uncertainty doubt by raising an objection without solid evidence that it explains what is observed. The latest salvo is from UncleJohnReturns and I’ll cover that in my next article.

Attack references

So you can see their arguments first-hand and decide for yourself:

1. Real Truther and be sure to see this post showing how he keeps changing the topic

2. Professor Jeffrey Morris posts

Introduction

We know the COVID vaccines kill people. The question is by how much?

A Japanese paper from 2022 said this and this is just one type of death from the shots:

Myocarditis mortality rate ratios (MMRRs) and their 95% confidence intervals (95% CIs) after 11 receiving SARS-CoV-2 vaccine compared with that in the reference population (previous 3 years) 12 were higher not only in young adults (highest in the 30s with MMRR of 7.80) but also in the elderly. 13 Standardised mortality ratio (SMR) for myocarditis was 1.35 (0.84 to 2.55) for those 60 years or 14 older and 1.69 (1.18 to 2.42) in overall age. The risk of myocarditis mortality in the SARS-CoV-2 15 vaccinated population may be 4 times or higher than the apparent MMRRs considering healthy 16 vaccinee effect. Unreported post-vaccination deaths should also be considered as suggested by the extremely high myocarditis mortality odds ratio (148.49; 89.18 to 247.25)

And then there’s this new Italian paper where they reanalyzed previous data and came to the opposite conclusion: the COVID vaccines are not safe: “The multivariate values were 2.40, 1.98, and 0.99” for the all-cause death hazard ratio for those getting 1, 2, and 3 shots.

And then there are all those death or serious adverse event reports which are up to 50% higher for Moderna than Pfizer: VAERS, yellow card, clinical trial, V-safe, etc.

What do you think the most likely explanation is?

According to Professor Jeffrey Morris, all this data is consistent with a safe and effective COVID vaccine and that’s the best fit to the observed data.

I couldn’t disagree more.

At least they do agree that Moderna has higher mortality after someone gets the shot, but they are convinced that every single datapoint on that was simply that Moderna was distributed to the people with higher comorbidities for every age in every country.

And they want you to believe that the Italian study where they actually corrected for comorbidities and still got a 2:1 higher odds of death in the COVID vaccinated… they want us to ignore that paper and just trust them… and that its just that people who were more likely to die always got Moderna. And if you were even more likely to die, you got yet another brand. They are all safe.

Argument #1: OK, you’re right. Moderna has higher mortality but that’s because it’s less effective in preventing COVID deaths, not because it is increasing ACM.

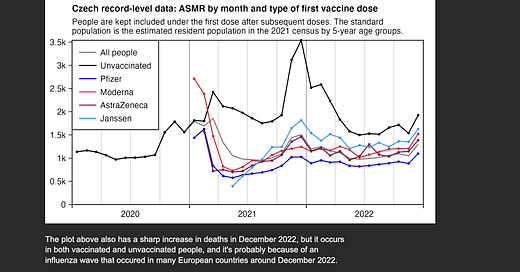
This is easily debunked using the chart that the Real Truther used to demand I retract my analysis which he lifted from Henjin’s analysis:

Figure 1. The gap between Moderna and Pfizer is steady, even when there is no COVID. The Real Truther didn’t notice that. Now he wants to run away when I ask him to explain how that is safe.

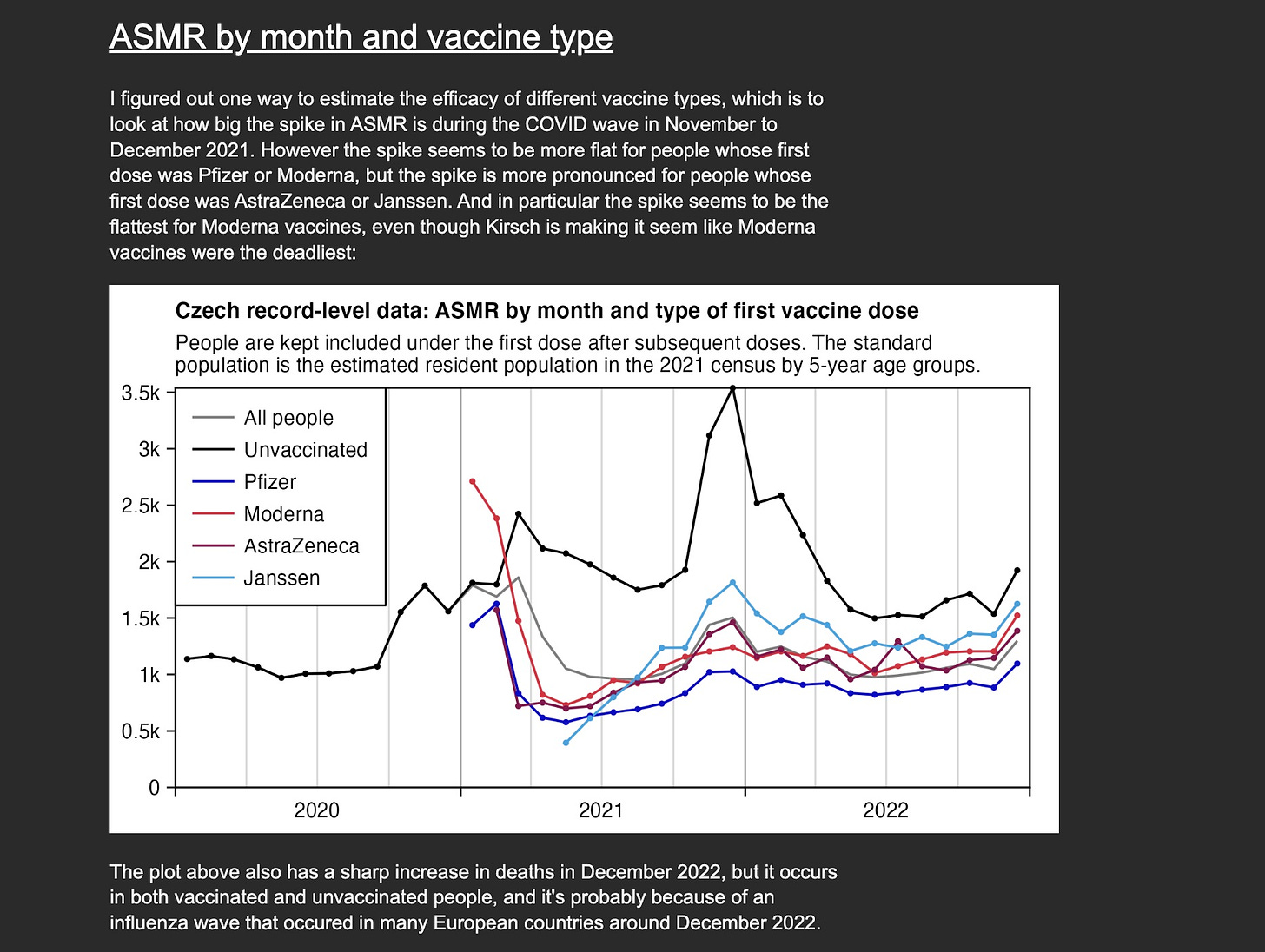
The blue is Pfizer. Red is Moderna.

Truther never bothered to check the dates when COVID deaths were near zero in the Czech Republic. But I did.

And guess what?

The distance between the curves remains steady during times of very high COVID and times of no COVID.

That means two things:

1. Moderna is 50% more deadly than baseline ACM (due to the separation during no COVID times). So Truther inadvertently proved Moderna is a train wreck. When I asked him to explain how there could be an ACM differential during no COVID, he wanted to talk about something else.

2. The vaccines were not substantially different in their ability to prevent death. If one were more effective than the other, we’d see the gap narrow or widen during COVID. Which could mean that they worked really well, worked half way, or didn’t work at all.

The bottom line is that because Moderna’s mortality rate was 50% higher during non-COVID times, it means that the Moderna vaccine increased baseline ACM by 50%. That’s a disaster because vaccines are always supposed to reduce ACM, not increase it.

Oh, and I checked this independently as well. See d2-jun21-deaths-filtered-by-month.xlsx in the repo analysis directory: during no COVID periods the gaps were just as wide.

So thanks to The Real Truther, we can now show the mortality differences were not due to vaccine effectiveness, but were 100% due to increasing ACM from baseline ACM.

This is a train wreck and The Real Truther helped me prove it! Poetic justice.

This is extremely damaging because they can’t explain why there is a difference when there is no COVID.

Nobody’s going to believe the shots reduced ACM. All these funerals that people are going to are of the vaccinated. Check out Jay Bonnar’s friends, all vaccinated, all died unexpectedly, 4 died on the same day as the shot.

Is it possible these shots reduced COVID ACM by equal amounts? Yes, but very unlikely. We have other data like the JAMA VA study and the US Nursing home data that show COVID mortality isn’t improved.

Finally, a 50% differential is just way too big for COVID for younger ages. My Czech friends tell me you can get to 12% if you were 100% effective, no way you can get to 50%.

But since there’s a differential at no COVID, it ends the debate.

Argument #2: The vaccinated have half the all-cause mortality as the vaccinated. The vaccines are saving lives!

Here’s the attack:

We indeed see that as @stkirsch notes, that Moderna has substantially higher death rates that Pfizer. However, Moderna still has lower death rates than unvaccianted, and not out of line with background death rate for the age groups. So when considered in context of all vaccines, unvaccinated, and background death rates, the key result is Pfizer has lower death rates that the rest. Not sure how he gets that Moderna is "killing many people" and needs to be "removed from the market"

…

So when considered in context of all vaccines, unvaccinated, and background death rates, the key result is Pfizer has lower death rates that the rest. Not sure how he gets that Moderna is "killing many people" and needs to be "removed from the market"

and this comment:

How can you say these data prove Moderna is "a kill shot" when their death rate is less than it is for unvaccinated, and not greater than the actuarial baseline death rate in the population from 2015-2019?

The thing they NEVER point out is that the mortality difference between the brands is the same during no COVID and high COVID as we saw from Fig. 1 above. So the mortality difference can’t be the vaccine saving lives since that only happens during COVID. So the mortality difference must be due to an increase in ACM of Moderna vs. Pfizer. Even if Pfizer is 100% safe, a mortality difference during non-COVID periods proves, without a doubt, that Moderna increases baseline ACM by as much as 50% or more.

As to why the vaccinated have lower mortality than the unvaccinated is simple and we see it all the time: selection bias also known as the healthy vaccinee effect (HVE) which Professor Morris knows very well about but fails to mention in his argument.

This was reiterated in a Japanese study and in other papers (including the HVE papers in my previous article):

The paper goes on to point out during one flu season, people who got the flu shot had a 64% lower mortality than their unvaccinated peers. The flu shot itself provides no ACM benefits. If it did, people with multiple flu shots would live almost forever and the reduction would be the same every flu season.

So it’s totally normal for the two groups to have different mortality and this is well known.

If the COVID shot itself lowered their ACM by 50% or more like the flu shot appears to do, then everyone would be lining up to get the COVID shot every month to maintain their relative immortality.

People who opt for the shots have higher socio-economic status and are not as likely to opt for a shot if they are going to die.

That is why there is a difference when there is no COVID: the groups are different.

And this is precisely why comparing brands of those who opt for vaccination is the single best real-world comparator of safety: because everything is similar except for the brand that they were assigned by the vaccinator based on what was at hand, not based on a comorbidity questionnaire. And while convenience sampling can create biases for example for a single age and sex, the biases are generally small and they are evenly distributed so that if one age/sex had higher comorbidities, another group would have lower so that at the end of the day, the differences within a given sex/age group should be small.

So comparing with the unvaccinated is not the right comparator and is a diversion.

Professor Morris knows this about the unvaccinated, but most other people don’t, so he can use it to fool people. A lot of the vaccine studies get away with this trick as well, such as this one published by the CDC (FDA discovers fountain of youth) showing dramatic reductions in ACM caused by the shot (when it was really caused by the selection bias).

Argument #3: This is all from bias in the convenience sampling. Both vaccines are very safe.

In the absence of any evidence of a systematic bias based on comorbidities, the most probable estimate is even distribution of people with high comorbidities between each brand since there is nothing that would favor one brand over the other.

So if this were due to convenience sampling, and if there are 80% Pfizer shots and 20% Moderna shots, we’d expect 20% of the high mortality people would get assigned to Moderna and 80% to Pfizer and the ratios would average out at the end of the day with some age groups favoring Moderna, and approximately an equal age ranges favoring Pfizer. The ratio can be lopsided, but the more lopsided it is, the less likelihood it is that this explains the effect.

This hypothesis doesn’t match the data at all which is why I rejected it as a plausible explanation.

Virtually all the MRRs are >1 and there are no strong MRRs in the opposite direction. So we have to discard that hypothesis since it just doesn’t fit the observed data in this study or other studies.

In more detail for those having a tough time understanding what I just wrote.

There are two fundamental hypotheses available:

1. Moderna is 50% more deadly

2. Convenience sampling caused the discrepancy which makes it looks like Moderna is deadly, but it’s just bad luck.

So I looked at the data to decide whether it is 1, 2, a combination, or neither that fits the data.

If for each age group, the MRR was between 2 and 1/2, then it’s pure convenience sampling and I would have picked that.

But if for each age group, the MRR was 1.5 or some other fixed value, I’d opt for explanation #1 and reject #2 especially since I also have data from FIVE other studies showing a consistently higher adverse event rate for Moderna (v-safe, VAERS, Fraiman study, Yellow card stats, and mRNA active ingredient amount). It all fits like a glove. What are the likelihoods of 5 other independent methods all getting unlucky and assigning all the high comorbidity people to Moderna? Astronomically small.

Also, if convenience sampling bias was the Soule explanation for the observed data, then we wouldn’t be able to explain the 8% rate of people requiring medical care reported in the V-safe database. But if we took the other hypothesis, we’d have something that’s totally consistent with the v-safe data.

The bottom line is that the data was a near exact fit to hypothesis 1 which is why I proposed it and rejected hypothesis 2.

Obviously Professor Morris thinks it is the opposite and we’ll just have to disagree on that one.

Confirmation for hypothesis #1

We have confirmation from v-safe that Moderna, on a per shot basis, produced significantly more serious adverse events. You can see from the dashboard that Moderna has more serious events reported than Pfizer even though 40% or so of the shots were Moderna.

We have confirmation in VAERS as well as I pointed out in the main article.

We have biological plausibility for all this: the amount of mRNA was over 50% higher for Moderna as I’ve pointed out in the main article.

We have confirmation from the Fraiman study as I detailed in my original article.

And finally we have this nice note from Norman Fenton that the same was found in the UK.

So everywhere we’re seeing a greater number of adverse events caused by Moderna.

Professor Morris just can’t seem to accept that. He wants us to ignore that and prove that the effect cannot be due to sampling bias.

I just explained how unlikely that would be.

I’ve asked Professor Morris, “Why don't you show me a real life example where convenience sampling showed mortality rates were always higher in one brand in 40/40 single age groups which had enough data to make a reasonably good ratio?”

So there is nothing to support convenience bias as the most likely explanation, and everything consistent with “it’s a more deadly shot due to the higher amount of poison in each shot.” That’s why I reject the convenience sampling bias argument: the data and the evidence just doesn’t support it at all.

The real life example of convenience sampling provided by Professor Morris as proof he is right actually is proof he is wrong!

Consider this post:

which has this graphic:

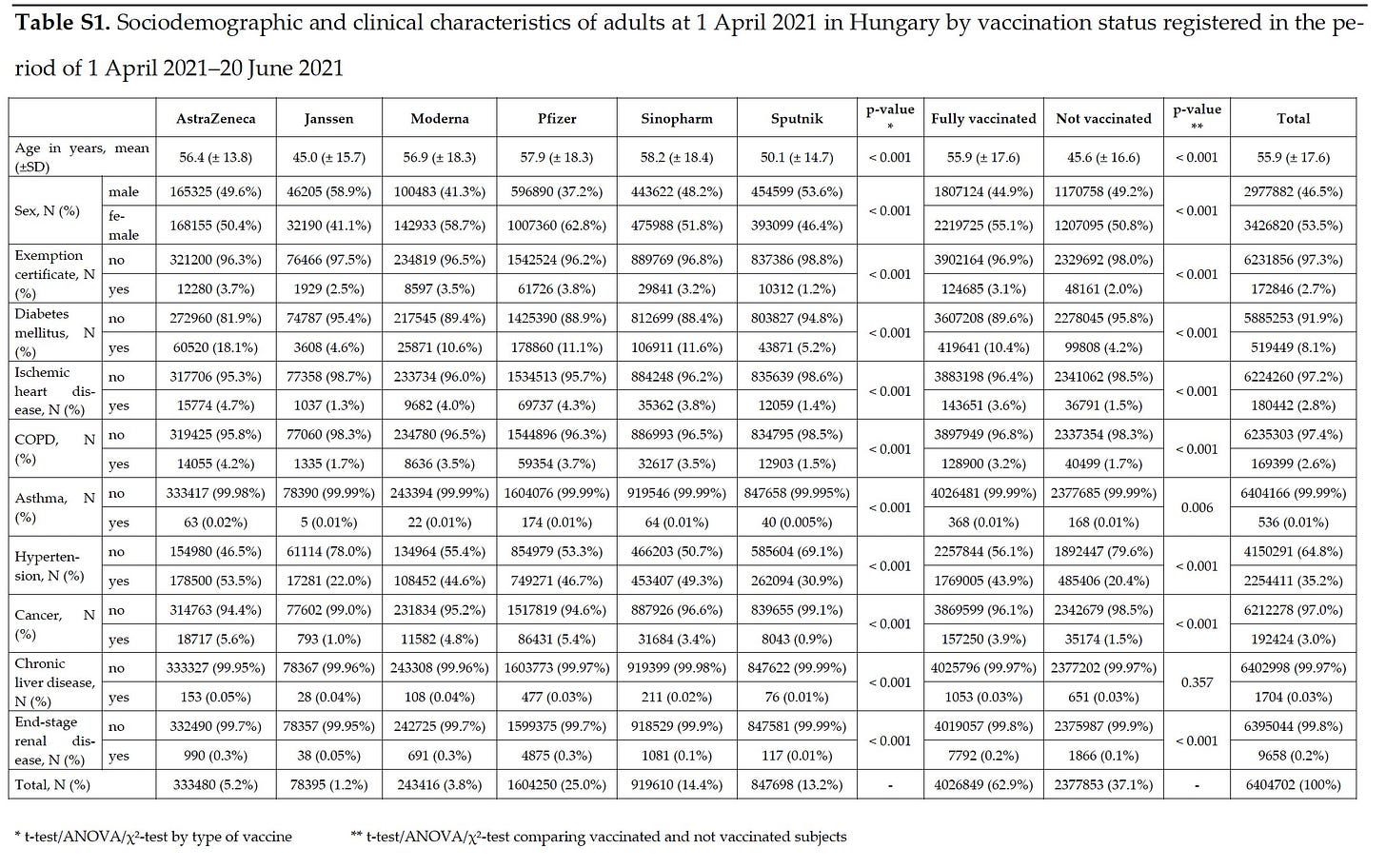
Professor Morris isn’t showing us than in every single digit age range for the same ages between the vaccine brands that all the Moderna people had 50% or more higher comorbidities than the Pfizer group. That would be very convincing. But he just shows a summary table.

The central limit theorem guarantees that the sample means form a normal distribution. There’s no getting around it. It’s the law of large numbers.

In plain English, it means that if we were to look at the comorbidities of Moderna vs. Pfizer for each individual age for the same sex, we’d find that, on average, half would be higher, and half would be lower. The chance we’d be able to pick 40 consecutive ages and have the combined comorbidities be slightly higher in Moderna is 1 in a trillion, the same probability as the chance of throwing a fair coin 40 times and getting all heads.

But we didn’t see a slightly higher effect in the groups of different ages. We saw a massive difference in the groups. The chance of 40 consecutive ages have comorbidities 50% higher for each sex and each age chosen is about as close to zero as you’ll ever see.

Now we didn’t see 50% in all 40 out of 40. But you get the idea. We saw an increase in 40/40, and a good chunk were 50% or more.

So why does Professor Morris ask me why did I reject the convenience sampling bias hypothesis? Two possibilities:

1. he’s never heard of the central limit theorem

2. he’s trying to gaslight me

3. he doesn’t understand convenience sampling w.r.t. the central limit theorem

So in his own example above, what do you think the chance is of Moderna having over 10% higher (or lower) comorbidities then Pfizer in every single one of the 10 categories listed is? Let’s take a look at the actual data for Pfizer and Moderna. We only have the averages here so we can compare apples to apples (i.e., same age and sex in each group). But we find only two categories where the difference is >10%:

1. cancer: 12% lower

2. chronic liver: 33% higher

But the chronic liver difference is on a very tiny absolute difference.

So has Professor Morris demonstrated to you that the Moderna cohort can easily have a 50% higher (or lower) likely all-cause mortality for a large range of single age/sex category?

Nope. He’s shown that you can’t violate the central limit theorem.

And the central limit theorem guarantees he’s wrong.

Is it possible that for one age/sex, we might see 50% higher comorbidities? Absolutely!

Is it possible that for another age/sex combo, we might see 50% lower comorbidities? Absolutely!

Both are exactly the same probability in the absence of a systematic bias.

So again, finding such a huge increase so consistently and without a single example in the other direction is extremely unlikely.

In short, the biases we observed were too large and consistent to be explained by convenience sampling bias due to the central limit theorem.

Could there be a systematic bias where people were asked what their comorbidities were on a form and then only assigned Moderna if there were over 5 and AstraZeneca if the number was over 10? Absolutely!!!!

But there is no evidence of that and none of the attackers haven’t asserted that any such systematic bias was present.

Basically, their argument is “How can you possibly assume that the dice is anything but a fair dice?” And my answer is: “Well, I rolled it 40 times and each time it rolled 6, so I’m pretty sure the dice are loaded.”

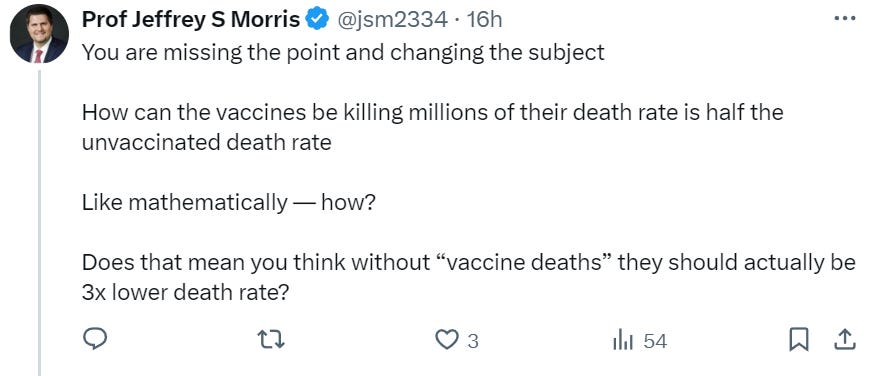
Argument #4: How can the vaccines be killing millions of their death rate is half the unvaccinated death rate Like mathematically — how?

Happy to provide the mathematical example. It’s not really hard.

Say you have a population of 100 people. 5% have don’t get vaccinated and have an average ACM of 2,000.

95% have an average ACM of 1,000 (deaths per 100,000 person years) which is a pretty typical crude mortality rate for a large population (healthier/younger populations might be 800 or lower). After the shots, for the old people who have the greatest number of deaths, the ACM shoots up by 10% on average (lower for older people, higher for younger people) and stays elevated.

Then you’ve narrowed the gap between the two groups so it’s now slightly under a factor of 2. But for the people likely to die you’ve increased their mortality by 10%.

In the US, if we increase the mortality of the people who are the most likely to die by 10% on average, that will generate 320,000 excess deaths per year. The vaccine has been out for 3.5 years. So you can get over 1M deaths and still have the vaccinated die at half the rate of the unvaccinated.

In the case of the Czech Republic, you can see from Figure 1 above (that THEY provided and reference as definitive truth), that the mortality gap between the vaccinated and vaccinated narrows at the right end of the graph.

There are only three ways to get a narrowing between the two separate groups. Either the unvaccinated suddenly got a lot healthier or the vaccinated are dying at a higher rate or there is a combination of both effects. We don’t know of anything that might cause the unvaccinated to be healthier. But for the vaccinated, we have a smoking gun called the COVID vaccine. So what changed is the vaccinated are now dying at a higher rate and the most likely cause of such a large event is either the vaccine, something very highly correlated to getting a vaccination. Having such a large mortality increase over wide age groups leave us with one solution it was the vaccine. If it wasn’t, then I’m open to data showing it wasn’t but hand waving attacks about mathematical impossibilities are simply not going to be persuasive for an alternative hypothesis.

And the claim that this is age confounded is false because the graph they are promoting is ASMR which is age standardized mortality rate.

And the claim the earlier disparities were due to COVID doesn’t hold water either because you can measure the gap after the big 2021 COVID wave dropping to zero in around March 2021 and compare that to the gap at the end.

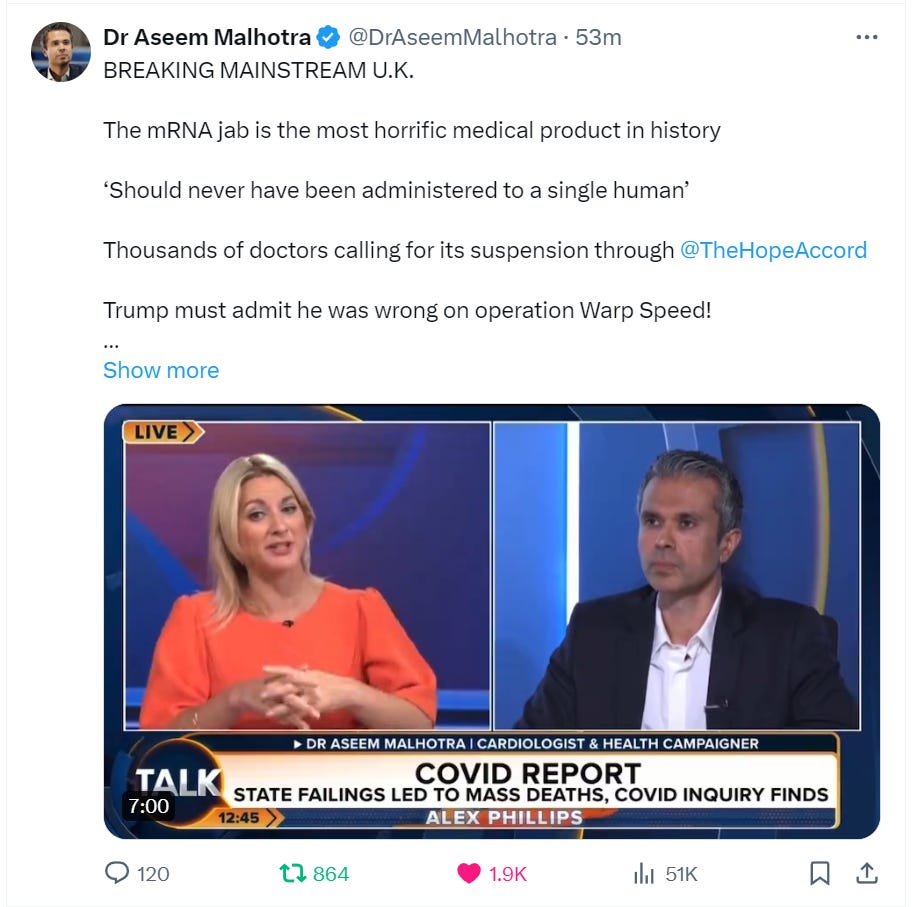
Consider that thousands of doctors are calling for a halt to these shots because they see how deadly they are like my friend cardiologist Aseem Malhotra who lost his father from the COVID vaccine:

And we have large scale independent surveys of the American public showing that people knew as many people dying from the shots as from COVID.

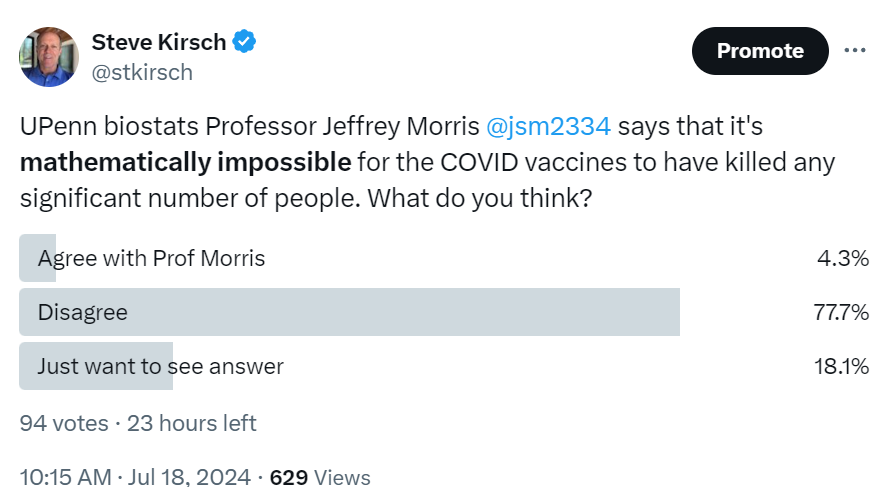
Yet we have a UPenn Professor of Biostatistics claiming that that deaths from the COVID vaccine are a mathematical impossibility. You can’t make this stuff up. That’s why I screenshotted his post above.

Either all those people who died are still alive, or Professor Morris is very bad at math. Which do you think is more likely? Here’s what X users thought:

Argument(s) #5 from “The Real Truther”

In his post and his follow up post, thereal_truther claims that:

1. We have no way to know if the Czech comorbidity distribution of the various vaccine brands is anything like the Hungary distribution which shows massive differences outside of age.

2. We know Moderna's death rate in your data is lower than the unvaccinated and lower than the baseline death rate.

3. Because of this we can theorize that any differences between Moderna & Pfizer are likely related to who received the vaccine (and where) rather than the vaccine being the driver in mortality differences.

4. Until you can show who got each vaccine and where they got it factoring in known health factors and comorbidities you cannot in good faith continue to claim the Moderna vaccine is causing mortality.

5. The vaccines didn't cause anyone to die at any higher rates than the actuarial baseline death rate in the population from 2015-2019.

6. If you look at the ASMR during the COVID wave in December 2021 with the ASMR in July 2021, Moderna MR was only 1.3X higher and Pfizer was 1.5X higher so clearly Moderna provides a 20% higher relative mortality benefit during COVID than Pfizer does so the Moderna shots provide a COVID death benefit.

I’ll respond to each of his claims, but this is getting tiresome because Truther just doesn’t read anything I post to dispute his claims:

1. As I pointed out above, the Hungary distribution didn’t show a systematic bias between Pfizer and Moderna. See the “The real life example of convenience sampling provided by Professor Morris as proof he is right actually is proof he is wrong!” section I wrote earlier. I’m happy to clarify any sections if it’s not understandable.

2. I agree! I pointed out in Argument #2 why the unvaccinated are not a useful comparator. And the gap got narrower over time which means the vaccinated got sicker relative to the unvaccinated. How did that happen? See Argument #4 above where I explain this in great detail.

3. No, as I explained in Argument #1 above. The disparity exists when there is no COVID. Vaccines only can reduce ACM when a disease is present. If there is an ACM difference when there is no disease, at least one of the vaccines is too dangerous to use.

4. See Argument #3 above. The central limit theorem means biased convenience sampling just doesn’t explain the observed data. The hypothesis just doesn’t match the data. I’m sorry this is not working out for you.

5. That may be true, I haven’t looked at that, but it’s mostly true since the vaccinated have half the death rate of the unvaccinated. But this is not caused by the vaccine as everyone knows. See Argument #2 above and I suggest you read the Japanese paper I linked to. If you have any questions after you’ve read and understood the paper, please let me know.

6. I see that, but the ratios ebb and flow. It would be very impressive if Moderna “closed the gap” during COVID waves, but it never closes the gap. While I am overjoyed that Moderna might provide a mortality benefit, if you want to make that claim you need to show me the 95% confidence bands on that difference and also show consistency that for every time there is a COVID wave you see a narrowing of the same amount and that the narrowing is within the 95% confidence interval. I showed the 95% confidence intervals when I made my assertion in my original document. So if you are going to make claims like that back it up because these ratios can have statistical noise that can make these observations interesting but not statistically significant. And even if you were to prove that there was a benefit, it is simply irrelevant because increasing ACM by 50% is not worth a 20% lower risk of the tiny chance I’d die from COVID. You should pay attention to the posts of people you quote in your posts like Jean Fisch. You reposted this post she wrote where she cherry picked the <60 data and assumed that COVID deaths were caused by COVID, so you must trust her. But did you realize she also posted this post where COVID’s affect on ACM is likely between 0 and 5% which I actually don’t disagree with; it’s certainly in the ballpark. So why would you take a vaccine which increased your ACM by 50% for a 1% mortality benefit?? It’s 1% because the 5% max impact is reduced by 20% by Moderna and 5% of 20% is 1%.

It’s frustrating Truther keeps rehashing arguments that I’ve already addressed and doesn’t appear to read anything I write.

Arguments #6 from Professor Morris (via X DM)

On Jul 18, 2024, he wrote:

Again, not sure how "convenience sampling" is relevant here given we are talking about entire-population data with no sampling done.

And can you explain to me how you think I am trying to "gaslight" you and how the central limit theorem is relevant to the simple points I've been trying to make, that you can't wish away confounders and that the death rates for the "killer" Moderna shot are less than the death rates observed for unvaccinated periods or from historical baseline rates?

The vaccines in Czech Republic (CR) were distributed based on availability. So this is not randomized on a per person basis; it’s more or less “chunk randomized” since the vaccines were delivered in batches. You got whatever was available when you went in to be vaccinated. So you could call it “cluster randomized” or “convenience sampled.” There was no systematic bias in CR for who got what brand.

A huge pile of Moderna could be delivered to an elderly care home for very sick patients. But it’s equally likely (normalized for total doses given) that it happens for Pfizer.

So if the effect was caused by distribution bias, you’d see age ranges where Pfizer was “safer” and age ranges where Moderna was safer. But typically, those differences would be minor. At the end of the day, with sufficient numbers of recipients, it would be unlike to have a substantially different number of wins for one brand or the other.

In short, the central limit theorem says that the number of wins is a normal distribution with a mean in the center where there is a an equal number of wins.

Now if Pfizer wins the MR ratio in nearly every single age category, for both male and female, then it’s really unlikely Pfizer just got lucky.

It’s like if Pfizer flips a fair coin 50 times and get heads. And Moderna flips the same fair coin 50 times and gets 50 tails in a row. While this is certainly statistically possible, the odds of this being observed are extremely low.

I’ve explain this in more detail in Argument #3 (read the whole section up to Argument #4).

Pfizer is nearly as unsafe as Moderna on a per shot basis

The Czech data shows, that at best, Pfizer is completely safe and Moderna is a disaster.

But you only need one look at the V-safe data to know that Pfizer cannot be safe.

If the Pfizer vaccine was safe, we wouldn’t have an 8% serious adverse event reporting rate in V-safe, something the government tried to hide from us.

If Pfizer was safe, nearly all these V-safe reports would be from Moderna.

But they aren’t. A slight majority are from Moderna, even though Modera was 40% of the vaccine delivered. Meaning Moderna had an event rate that was over 50% higher than Pfizer’s.

So make no mistake… both vaccines are deadly.

These people simply cannot explain the v-safe data if the vaccines are both safe. It is inconsistent with their belief system so they come up with some excuse to ignore it.

That’s bad science. If you have the right hypothesis, you can explain all the data.

Final argument (courtesy of Clare Craig)

All the other vaccines had nearly all their datapoints worse than Pfizer.

If the vaccines are equally safe, then such consistency on a per brand basis for the “it was an artifact of convenience sampling” hypothesis is simply unprecedented in human history.

A claim that Pfizer was given to the particularly healthy somehow is not supported by any evidence. We can't see how that can happen and still result in similar disparities in terms of mortality consequence in every age group by brand.

How Professor Morris can claim that this is a plausible scenario is troubling.

Summary

All the people attacking my analysis are not interested in the truth. If they were, they’d be calling for data transparency.

They keep trying to poke holes in the argument hoping one will stick.

They tend to change the topic when challenged on their attacks. And they avoid answering pointed questions such as you see here where “Truther” keeps wanting to change the topic when he can’t answer a simple question that proves he’s wrong.

The single best fair comparison we have today is the mortality comparison by brand. That’s as close to ideal that you’ll ever get in the real world. Yet they want to distract your attention from that.

And nobody wants to see or compute this number for other countries. Why not?

Why aren’t any of the attackers calling for data transparency?

They want you to focus on the vaccinated die at half the rate of the unvaccinated, and want you to ignore the fact that it happens when there is no COVID around. Which means it’s an effect not related to the safety of the vaccines.

They just can’t accept the data in plain sight. They are deliberately trying to mislead people into believing a fairy tale. Don’t fall for it.