The New Zealand data released by Barry Young can be used to prove the COVID vaccines are unsafe

I'll show you the easiest way to do that in this article. However, it doesn't appear that any of the New Zealand lawmakers or health authorities are interested in what the data shows.

Executive summary

Recently, I took another look at the New Zealand data leaked by Barry Young.

It turns out it is trivial to show that the 1 year mortality from the time of the shot is batch dependent, varying by a factor of 2 or more. That’s a huge problem for the mean mortality rates to have such a huge variation.

The other important realization (that I’m apparently the first person to point out) is that for a given age range and vaccination date, if you do a histogram of the mortality rates of the batches, if the vaccines are safe, these will form a normal distribution because of the central limit theorem. That simply doesn’t happen. So that’s another huge red flag that the vaccines are not safe.

The code and the data

The code for the New Zealand batch analysis was trivial to write. It can be found in my NewZealand Github.

Batches vary by over 2X in mortality rate, even if they are given in the same month to the same age range of people

You can’t have a 2X variation in the 1-year mortality rate for a given vaccination date and 5 year age range. That suggests that the vaccine is not safe. We can do a Fisher exact test to prove our point.

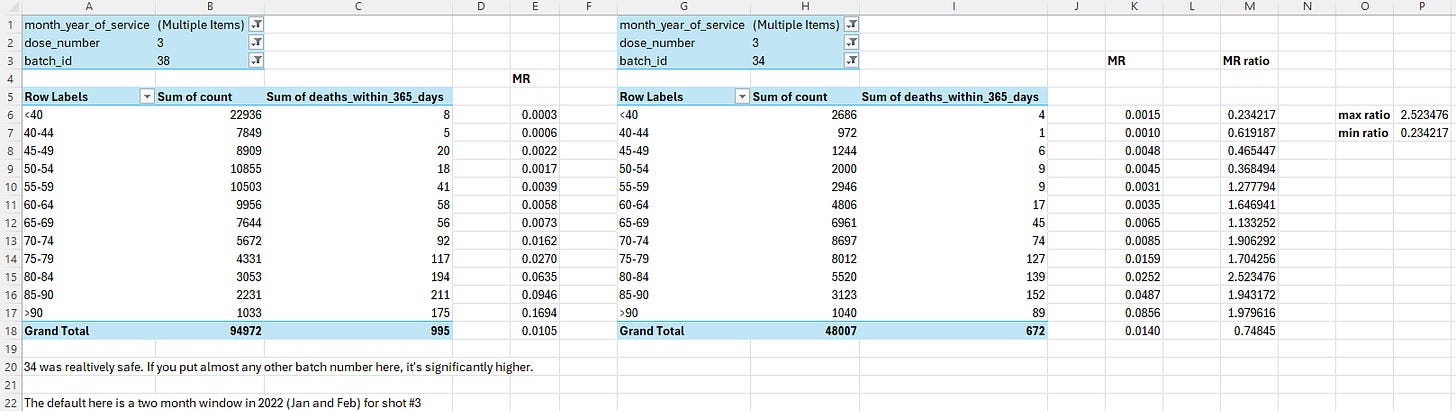
Let’s take the 80-84 year old line because there are lots of deaths there so it’s easier to show the differences between the batches are statistically significant (which should never happen if the vaccines are all safe).

Statistics for batch 34 vs. batch 38 for ages 80 to 84 given Jan 1, 2022 to Feb 28, 2022.
The Fisher matrix is (5381 2859 139 194 8573).

One-sided p-value 7.956587575710554e-18
Max likelihood estimate of the Odds ratio= 2.6265352736010104
95% Confidence Interval(low=2.091, high=3.305)

So we’re done. You can try values higher than 34 and you’ll see this wasn’t cherry picked. The 2X variation is easy to find and is statistically significant when we have enough data in the batches.

The distribution of mortality rates

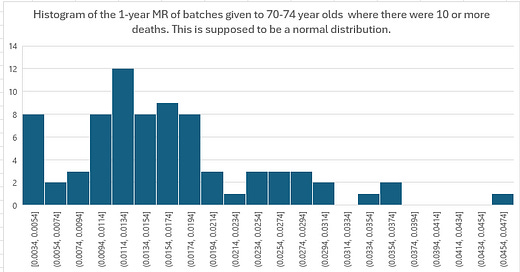
The central limit theorem guarantees that if we make a histogram of the mortality rates of each batch for a given age range, it must form a normal distribution if the vaccines are safe (all other things being equal).

All other things aren’t equal in the real world because a few batches are distributed primarily in areas with a high Maori population, but we should we a very strong peak for most of the 58 qualifying batches.

Let’s look at 70-74 year olds for all batches given on or before September 2022. The cutoff date allows people 1 year to die. I picked this age range because there are 58 batches that with 10 or more deaths, so we should have a very nice looking normal histogram.

Histogram of 1 year MR rates for 70-74 year olds. The y-axis are counts, the x-axis is the MR bucket. It’s not a normal curve so we are not measuring a single population mean value as we expected to see if the batches are safe. This means the batches have their own excess mortality.

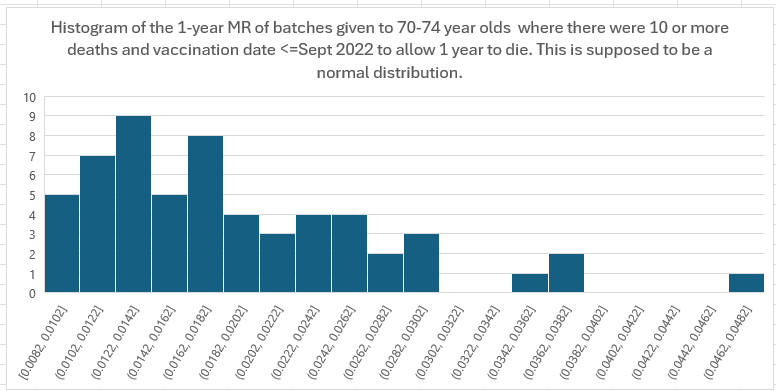
For reference, here’s what a normal distribution looks like for 60 data points randomly picked from a normal distribution with mean 0 and std dev 1. As you can see, you don’t see a lot of values after 2 standard deviations from the mean.

In the case above, we only looked at samples of 10 or more deaths so we’re looking at a standard deviation of around 30% at worst( 10 death counts) to under 10% at best (100 death counts). Per the normal curve, seeing 3 the std deviations (of 30% or less) should be rare, so we should rarely see any bars higher than twice the mean which is 2*.0148=.03. And yet we do.

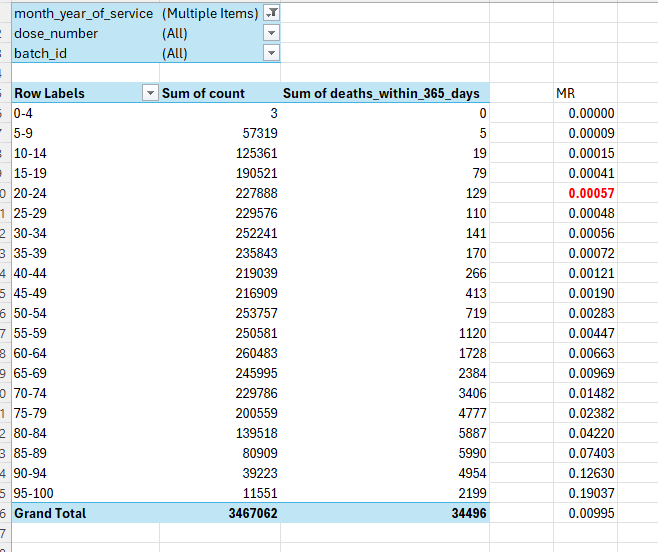
A third aspect: the spike in 20-24 year olds who have been vaccinated

This death spike in the 2024 age group should not happen. This is statistically significant. This doesn’t mean the vaccine is safe for the other age groups. It means that the safety signal is so large for the 20-24 year old age group that it sticks out like a sore thumb.

Summary

There are no published studies of the New Zealand data showing how it is consistent with a safe vaccine. That’s because the data shows otherwise.

But nobody wants to know that they were responsible for killing people. So they’ll ignore looking at the data for as long as they can get away with it.

Thank you to Barry Young for exposing this data so it can be analyzed and so we can inform people with an open mind of the harms.